Temperature dependence of the excitation spectrum in the charge-density-wave ErTe₃ and HoTe₃ systems

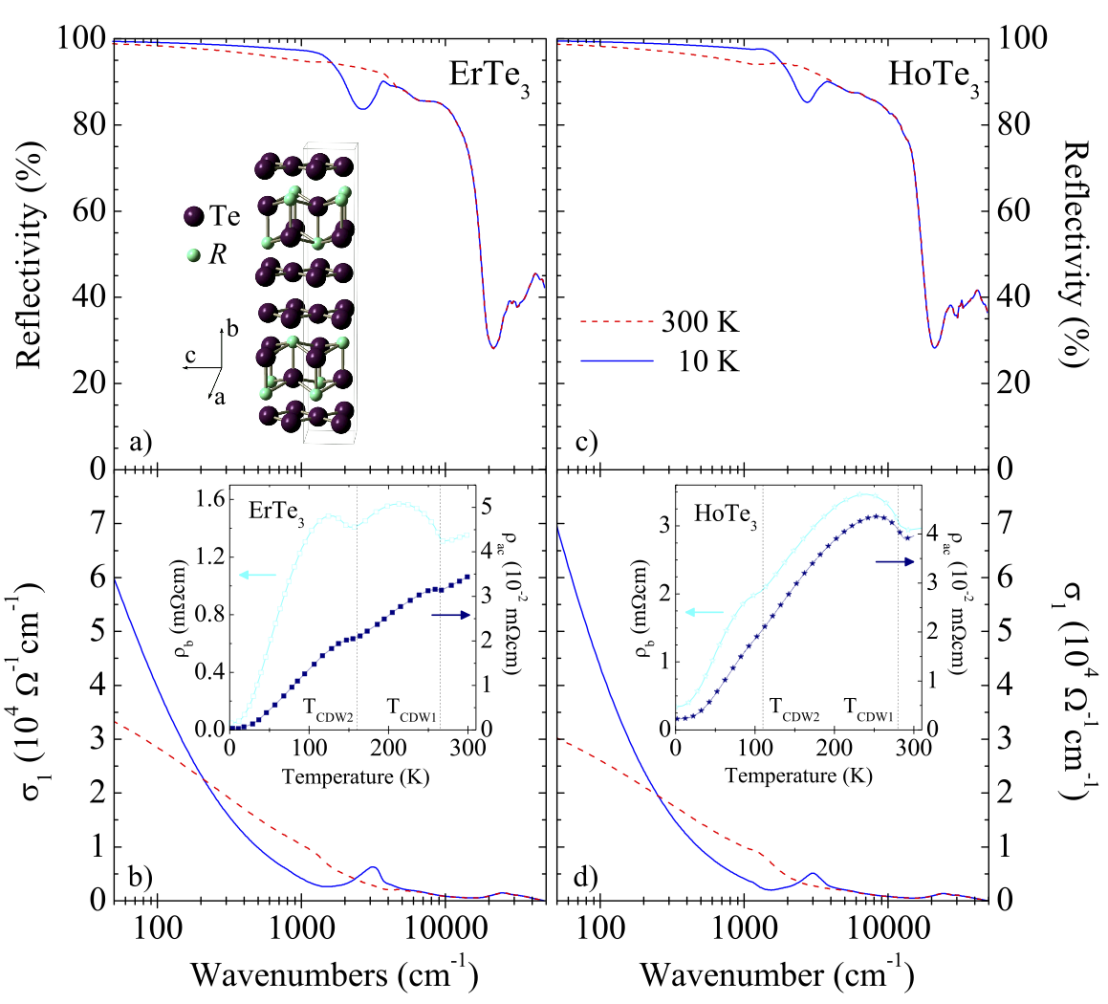
Recently, we focused our attention on HoTe₃ and ErTe₃, as representative members of the heavy rare-earth tri-tellurides (inset Fig. 3.14a) with transition temperatures (T_CDW1 and T_CDW2, both below 300 K) into the uni- and bidirectional CDW states, respectively (insets Fig. 3.14b-d). This allows us to have access to the temperature dependence of the relevant energy scales (e.g. the CDW gaps), on which very little was known. We compared the temperature dependence of the CDW phase transition with the previously studied impact of the lattice compression and of the pressure induced dimensionality crossover on the CDW formation.

Figures 3.14a and 3.14c display R(ω) for both compounds at 300 K (i.e., in the so-called normal state) and at 10 K (i.e. within the CDW states). At 10 K one can recognize the depletion in R(ω) at about 3000 cm^-1. These spectra are very much reminiscent of what has been seen in our previous studies on RTe₃ as a function of chemical and applied pressure. Exploring such a large spectral range allows us to perform reliable Kramers-Kronig (KK) transformations in order to achieve the optical functions. The overall view of the real part σ₁(ω) of the complex optical conductivity at those two selected temperatures is shown for both compounds in Fig. 3.14b and 3.14d.